This blog is intended to spotlight the facts about India, that have been erased from history. India has been rich in culture, religion, tradition, science, literature and prosperity since ever it was found to be existed and before as well. We have been grown up without knowing our prosperity, which is a must. Indians should not lose their pride, uniqueness and whatever. Here are some facts which I came to know, that are masked from the world history.
History Of Indian Mathematics
Despite developing quite independently of Chinese (and probably also of Babylonian mathematics), some very advanced mathematical discoveries were made at a very early time in India.
Mantras from the early Vedic period (before 1000 BC) invoke powers of ten from a hundred all the way up to a trillion, and provide evidence of the use of arithmetic operations such as addition, subtraction, multiplication, fractions, squares, cubes and roots. A 4th Century AD Sanskrit text reports Buddha enumerating numbers up to 1053, as well as describing six more numbering systems over and above these, leading to a number equivalent to 10421. Given that there are an estimated 1080 atoms in the whole universe, this is as close to infinity as any in the ancient world came. It also describes a series of iterations in decreasing size, in order to demonstrate the size of an atom, which comes remarkably close to the actual size of a carbon atom (about 70 trillionths of a metre).
As early as the 8th Century BC, long before Pythagoras, a text known as the “Sulba Sutras” (or "Sulva Sutras") listed several simple Pythagorean triples, as well as a statement of the simplified Pythagorean theorem for the sides of a square and for a rectangle (indeed, it seems quite likely that Pythagoras learned his basic geometry from the "Sulba Sutras"). The Sutras also contain geometric solutions of linear and quadratic equations.
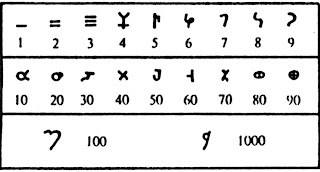
The Indians were also responsible for another hugely important development in mathematics. The earliest recorded usage of a circle character for the number zero is usually attributed to a 9th Century engraving in a temple in Gwalior in central India. But the brilliant conceptual leap to include zero as a number in its own right (rather than merely as a placeholder, a blank or empty space within a number, as it had been treated until that time) is usually credited to the 7th Century Indian mathematicians Brahmagupta - or possibly another Indian, Bhaskara I - even though it may well have been in practical use for centuries before that. The use of zero as a number which could be used in calculations and mathematical investigations, would revolutionize mathematics.
Aryabhata
Aryabhata was the first in the line of great mathematician-astronomers from the classical age of Indian mathematics and Indian astronomy. His works include the Āryabhaṭīya (499 CE, when he was 23 years old)[5] and the Arya-siddhanta. The works of Aryabhata dealt with mainly mathematics and astronomy. He also worked on the approximation for pi.
Aryabhata is the author of several treatises on mathematics and astronomy, some of which are lost.
His major work, Aryabhatiya, a compendium of mathematics and astronomy, was extensively referred to in the Indian mathematical literature and has survived to modern times. The mathematical part of the Aryabhatiya covers arithmetic, algebra, plane trigonometry, and spherical trigonometry. It also contains continued fractions, quadratic equations, sums-of-power series, and a table of sines.
The Arya-siddhanta, a lot work on astronomical computations, is known through the writings of Aryabhata's contemporary, Varahamihira, and later mathematicians and commentators, including Brahmagupta and Bhaskara I. This work appears to be based on the older Surya Siddhanta and uses the midnight-day reckoning, as opposed to sunrise in Aryabhatiya. It also contained a description of several astronomical instruments: the gnomon (shanku-yantra), a shadow instrument (chhAyA-yantra), possibly angle-measuring devices, semicircular and circular (dhanur-yantra / chakra-yantra), a cylindrical stick yasti-yantra, an umbrella-shaped device called the chhatra-yantra, and water clocks of at least two types, bow-shaped and cylindrical.
A third text, which may have 8 in the Arabic translation, is Al ntf or Al-nanf. It claims that it is a translation by Aryabhata, but the Sanskrit name of this work is not known.
Brahmagupta
Brahmagupta was an Indian mathematician and astronomer who wrote two important works on mathematics and astronomy: the Brāhmasphuṭasiddhānta (Correctly Established Doctrine of Brahma)(628) a theoretical treatise and the 'Khaṇḍakhādyaka a more practical text. There are reasons to beleive that Brahmagupta originated from Bhinmal.
Brahmagupta was the first to give rules to compute with zero. THe texts composed by Brahmagupta were composed in elliptic verse, as was common practice in Indian mathematics, and consequently has a poetic ring to it. As no proofs are given, it is not known how Brahmagupta's mathematics was derived.
In the Brāhmasphuṭasiddhānta verses 7 and 8 of chapter XXIV state that Brahmagupta composed this text at the age of thirty in Śaka 550 (=A.D. 628) during the reign of King Vyāghramukha, we can thus gather that he was born in 598[2]. Commentators refer to him as a great shcolar from Bhinmal, a city in the state of Rajasthan of Northwest India [3]. In ancient times Bhillamala was the seat of power of the Gurjars. His father was Jisnugupta.[4] He likely lived most of his life in Bhillamala (modern Bhinmal in Rajasthan) during the reign (and possibly under the patronage) of King Vyaghramukha.[5] As a result, Brahmagupta is often referred to as Bhillamalacharya, that is, the teacher from Bhillamala. He was the head of the astronomical observatory at Ujjain, and during his tenure there wrote four texts on mathematics and astronomy: the Cadamekela in 624, the Brahmasphutasiddhanta in 628, the Khandakhadyaka in 665, and the Durkeamynarda in 672. The Brahmasphutasiddhanta (Corrected Treatise of Brahma) is arguably his most famous work. The historian al-Biruni (c. 1050) in his book Tariq al-Hind states that the Abbasid caliph al-Ma'mun had an embassy in India and from India a book was brought to Baghdad which was translated into Arabic as Sindhind. It is generally presumed that Sindhind is none other than Brahmagupta's Brahmasphuta-siddhanta.
Although Brahmagupta was familiar with the works of astronomers following the tradition of Aryabhatiya, it is not known if he was familiar with the work of Bhaskara I, a contemporary.[5] Brahmagupta had a plethora of criticism directed towards the work of rival astronomers, and in his Brahmasphutasiddhanta is found one of the earliest attested schisms among Indian mathematicians. The division was primarily about the application of mathematics to the physical world, rather than about the mathematics itself. In Brahmagupta's case, the disagreements stemmed largely from the choice of astronomical parameters and theories.[5] Critiques of rival theories appear throughout the first ten astronomical chapters and the eleventh chapter is entirely devoted to criticism of these theories, although no criticisms appear in the twelfth and eighteenth chapters.
Bhaskara 2
Bhaskara 2 was an Indian mathematician and astronomer. He was born near Vijjadavida (Bijapur in modern Karnataka). Bhāskara is said to have been the head of an astronomical observatory at Ujjain, the leading mathematical center of ancient India. He lived in the Sahyadri region (Patnadevi, Jalgaon, Maharashtra).
Bhāskara and his works represent a significant contribution to mathematical and astronomical knowledge in the 12th century. He has been called the greatest mathematician of medieval India.[2] His main work Siddhānta Shiromani, (Sanskrit for "Crown of treatises,"[3]) is divided into four parts called Lilāvati, Bijaganita, Grahaganita and Golādhyāya.[4] These four sections deal with arithmetic, algebra, mathematics of the planets, and spheres respectively. He also wrote another treatise named Karna Kautoohala.
Bhāskara's work on calculus predates Newton and Leibniz by over half a millennium.[5][6] He is particularly known in the discovery of the principles of differential calculus and its application to astronomical problems and computations. While Newton and Leibniz have been credited with differential and integral calculus, there is strong evidence to suggest that Bhāskara was a pioneer in some of the principles of differential calculus. He was perhaps the first to conceive the differential coefficient and differential calculus.
Srinivasa Ramanujan
Srinivasa Ramanujan was an Indian mathematician and autodidact who, with almost no formal training in pure mathematics, made extraordinary contributions to mathematical analysis, number theory, infinite series, and continued fractions. Living in India with no access to the larger mathematical community, which was centred in Europe at the time, Ramanujan developed his own mathematical research in isolation. As a result, he sometimes rediscovered known theorems in addition to producing new work. Ramanujan was said to be a natural genius by the English mathematician G. H. Hardy, in the same league as mathematicians such as Euler and Gauss.He died at the age of 32.
Born at Erode, Madras Presidency (now Tamil Nadu) in a Tamil Brahmin family of Thenkalai Iyengar sect Ramanujan's introduction to formal mathematics began at age 10. He demonstrated a natural ability, and was given books on advanced trigonometry written by S. L. Loney that he mastered by the age of 12; he even discovered theorems of his own, and re-discovered Euler's identity independently. He demonstrated unusual mathematical skills at school, winning accolades and awards. By 17, Ramanujan had conducted his own mathematical research on Bernoulli numbers and the Euler–Mascheroni constant.
Ramanujan received a scholarship to study at Government College in Kumbakonam, which was later rescinded when he failed his non-mathematical coursework. He joined another college to pursue independent mathematical research, working as a clerk in the Accountant-General's office at the Madras Port Trust Office to support himself. In 1912–1913, he sent samples of his theorems to three academics at the University of Cambridge. G. H. Hardy, recognizing the brilliance of his work, invited Ramanujan to visit and work with him at Cambridge. He became a Fellow of the Royal Society and a Fellow of Trinity College, Cambridge. Ramanujan died of illness, malnutrition, and possibly liver infection in 1920 at the age of 32.
During his short lifetime, Ramanujan independently compiled nearly 3900 results (mostly identities and equations).Nearly all his claims have now been proven correct, although a small number of these results were actually false and some were already known.He stated results that were both original and highly unconventional, such as the Ramanujan prime and the Ramanujan theta function, and these have inspired a vast amount of further research.However, the mathematical mainstream has been rather slow in absorbing some of his major discoveries. The Ramanujan Journal, an international publication, was launched to publish work in all areas of mathematics influenced by his work.
In December 2011, in recognition of his contribution to mathematics, the Government of India declared that Ramanujan's birthday(22 December) should be celebrated every year as National mathematics Day, and also declared 2012 the National Mathematics Year.
Shakuntala Devi

Shakuntala Devi (November 4, 1929 – April 21, 2013), popularly known as "Human Computer", was an Indian prodigy mental calculator.
Shakuntala Devi was born in Bangalore, India, to an orthodox priestly family. Her father rebelled against becoming a temple priest and instead joined a circus, where he worked as a trapeze and tightrope performer, and later as a lion tamer and a human cannonball.[citation needed] Shakuntala Devi was only around three years old and she was roped in to help her father with card tricks. Her father left the circus and took her on road shows that displayed her amazing ability at number crunching. It is worth noting that she was able to do this, despite having had no formal education.[1] By age six she demonstrated her calculation and memorization abilities at the University of Mysore.[2] At the age of eight she had success at Annamalai University by doing the same. In 2006 she released In the Wonderland of Numbers which talks about a girl Neha and her fascination for numbers. She developed the concept of 'mind dynamics'.
Shakuntala Devi returned to India in the mid-1960s and married Paritosh Banerji, a senior IAS officer from Kolkata. The couple had a daughter, Anupama Banerji. Shakuntala Devi returned to Bangalore in early 1980s.
Conclusion
Here we come to an end.From reading this blog it is concluded that India had a glorious past in the field of mathematics. But today India lacks mathematician, not only India more mathematicians are required throuhout the world.There are many talented mathematician amongst us but there talents are wasted because they themselves dont know about it. There talents need a platform where they can produce it. I hope you enjoyed reading this blog. Please forgive me for any grammatical mistakes. I wrote this blog because I enjoy writting.Please give your precious comments and let me know if any improvement is needed. Thanks for reading. I hope it helped you.




No comments:
Post a Comment